
Next: Coordinates and parameterizations Up: 8.3 Vector Fields and Previous: Piecewise-smooth vector fields
The manifold definition given in Section 4.1.2 is often
called a topological manifold. A manifold defined in this way
does not necessarily have enough axioms to ensure that calculus
operations, such as differentiation and integration, can be performed.
We would like to talk about velocities on the configuration space ![]() or in general for a continuous state space
or in general for a continuous state space ![]() . As seen in Chapter
4, the configuration space could be a manifold such as
. As seen in Chapter
4, the configuration space could be a manifold such as
![]() . Therefore, we need to define some more qualities that a
manifold should possess to enable calculus. This leads to the notion
of a smooth manifold.
. Therefore, we need to define some more qualities that a
manifold should possess to enable calculus. This leads to the notion
of a smooth manifold.
 |
Assume that ![]() is a topological manifold, as defined in Section
4.1.2. For example,
is a topological manifold, as defined in Section
4.1.2. For example, ![]() could represent
could represent ![]() , the
set of all rotation matrices for
, the
set of all rotation matrices for
![]() . A simpler example that will
be helpful to keep in mind is
. A simpler example that will
be helpful to keep in mind is
![]() , which is a sphere in
, which is a sphere in
![]() . We want to extend the concepts of Section 8.3.1
from
. We want to extend the concepts of Section 8.3.1
from
![]() to manifolds. One of the first definitions will be the
tangent space
to manifolds. One of the first definitions will be the
tangent space
![]() at a point
at a point ![]() . As you might imagine
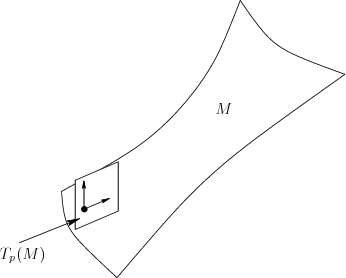
intuitively, the tangent vectors are tangent to a surface, as shown in
Figure 8.8. These will indicate possible velocities with
which we can move along the manifold from
. As you might imagine
intuitively, the tangent vectors are tangent to a surface, as shown in
Figure 8.8. These will indicate possible velocities with
which we can move along the manifold from ![]() . This is more difficult
to define for a manifold than for
. This is more difficult
to define for a manifold than for
![]() because it is easy to
express any point in
because it is easy to
express any point in
![]() using
using ![]() coordinates, and all local
coordinate frames for the tangent spaces at every
coordinates, and all local
coordinate frames for the tangent spaces at every
![]() are
perfectly aligned with each other. For a manifold such as
are
perfectly aligned with each other. For a manifold such as
![]() , we
must define tangent spaces in a way that is not sensitive to
coordinates and handles the fact that the tangent plane rotates as we
move around on
, we
must define tangent spaces in a way that is not sensitive to
coordinates and handles the fact that the tangent plane rotates as we
move around on
![]() .
.
First think carefully about what it means to assign coordinates to a
manifold. Suppose ![]() has dimension
has dimension ![]() and is embedded in
and is embedded in
![]() .
For
.
For ![]() ,
, ![]() and
and ![]() . For
. For
![]() ,
, ![]() and
and ![]() . The number of coordinates should be
. The number of coordinates should be ![]() , the dimension of
, the dimension of ![]() ;
however, manifolds embedded in
;
however, manifolds embedded in
![]() are often expressed as a subset
of
are often expressed as a subset
of
![]() for which some equality constraints must be obeyed. We
would like to express some part of
for which some equality constraints must be obeyed. We
would like to express some part of ![]() in terms of coordinates in
in terms of coordinates in
![]() .
.